A renowned metallurgist on his relationship with geometry, Japan, and more
My work has involved studying the geometries of materials such as crystals, glasses, twin boundaries, grain boundaries, and quasicrystals. Quasicrystals open new windows into the architecture of solids. Their beauty continues to attract me and I have worked on them for close to 36 years.
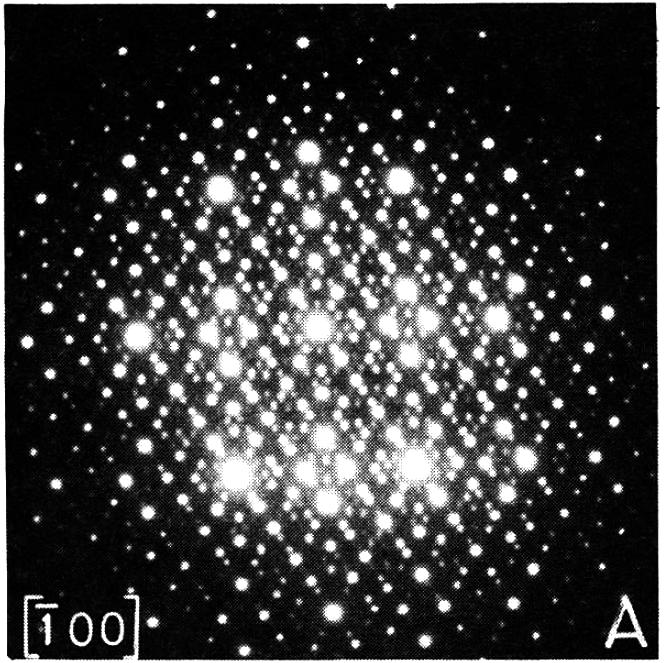
Quasicrystals or quasiperiodic crystals are important because they tell us how matter is organised. Scientists used to believe that solid matter existed in only two states – crystalline and amorphous. Crystals, especially minerals, have fascinated humans from early times. Their beautiful shapes were attributed to periodic and ordered arrangement of atoms. But quasicrystals mark a paradigm shift – they are ordered but quasiperiodic. They follow the Fibonacci sequence and can repeat a motif upto infinity, without being identical. We see examples of quasicrystals in Islamic art like the Alhambra palace in Spain, where geometry is used so beautifully. It has been shown that they can also occur in meteorites.
In 2006, a book that I co-authored with Eric A Lord and Alan Mackay, New Geometries for New Materials, was published. Soon after, it was translated into Russian, and most recently in December 2019, another translation was published in Japanese.

There were many influences that led to the point where I got involved in contributing to the book. I have been fortunate to have worked with a large number of mathematicians without any of them figuring out that I was not a mathematician myself! They could tell that I had a feel for it, perhaps. But the truth is that I did not study mathematics beyond Intermediate. I obtained my BSc (Hons) in Chemistry from the University of Madras, BE in Metallurgy at IISc, and PhD in Metallurgy from the University of Cambridge in the UK.
It was while I was at Cambridge between 1962 and 1965 that the book Introduction to Geometry by HSM Coxeter drew my attention. Coxeter is believed to be one of the greatest geometers of the 20th century, and his book was so lucid that I was able to clearly grasp what he said about concepts like polytopes – geometric objects extending polygons and polyhedra to higher dimensions. I even corresponded with him and was thrilled to receive a reply. From his work I learned that geometry links not just inorganic materials, but biological materials too, like DNA.
My first paper, “A field-ion microscopic study of the atomic configuration at grain boundaries in metals,” published in 1964, became a citation classic. In writing about the genesis of the paper, my doctoral supervisor at Cambridge, David G Brandon, made a reference to me having been inspired by Coxeter’s polytopes.
Brandon had also been supervisor to Dan Shechtman, the Israeli metallurgist who in 2011 won the Nobel Prize in Chemistry for the discovery of quasicrystals. In 1984, Shechtman published a paper on the quasicrystalline configuration in a metallic alloy. And nobody believed him. It took 30 years for the Nobel Prize committee to recognise his work. Shechtman and I didn’t just share a supervisor – we also share the same birth date, month and year! When I was a child, my parents changed my date of birth on paper by a few days so that I could gain admission to Loyola College, Chennai. I like to joke that if they hadn’t, I might have won the Nobel Prize too!
Dan Shechtman and I didn’t just share a supervisor – we also share the same birth date, month and year!
In addition to Coxeter, I was deeply influenced by articles in the Mathematical Recreations section of Scientific American by Martin Gardner, the English mathematician Roger Penrose’s 1974 paper on tiling which contributed to the eventual modelling of quasicrystals, and by the British metallurgist Cyril Stanley Smith, who emphasised that the information contained in the structure of materials is vital. In 1981, I returned to IISc where I collaborated with Kamanio Chattopadhyay in the Department of Metallurgy and we discovered decagonal quasicrystals. We published our first paper together in 1985, within months of the appearance of Shechtman’s seminal paper. Over the years, a number of gifted students, including Alok Singh who now works at the National Institute for Materials Science, Japan, also did their theses on quasicrystals under my supervision.
In 1987, I met Shechtman at a quasicrystal conference in Beijing. There, I also met the British crystallographer Alan Mackay. Numerous papers by Mackay on generalised crystallography and discussions with him influenced me tremendously. His 1981 paper on “De Nive Quinangula” predicted structures that traditional crystallography did not allow – like quasicrystals. This was reminiscent of Johann Kepler’s “De Nive Sexangula”, which in 1611 tried to describe why snowflakes have a hexagonal symmetry. It ignited my mind.
Back in Bangalore, I met Eric A Lord, a mathematician who was a visiting scientist at IISc, and had the good fortune of collaborating with him. Eric and I wrote a paper on Truchet tiles and another on the transformation of polyhedral grains. He translated several of my ideas, in particular those on quasicrystals and biological helices. His ability to visualise geometry and shape is unparalleled. Alan and Eric worked on periodic minimal surfaces and published several papers together.

The book New Geometries for New Materials came about because I was teaching classes at IISc on geometries – on intermetallics and so on. I took Eric’s help because I had the metallurgical concepts, but needed to put them into mathematical form. After all, geometry is all-pervasive in our studies of materials. Alan joined us too. Cambridge University Press published the book in 2006. Years later, Koji Miyazaki, from the Graduate School of Human and Environmental Sciences, Kyoto University, made the Japanese edition possible.
I have had a long and enduring relationship with Japan, beginning in 1966 when I was on a personal visit to Kyoto. In 1993, I was invited to Japan by Akihisa Inoue, a scientist at Tohoku University who made seminal contributions by inventing bulk metallic glasses. My collaboration with Motoko Kotani at Tohoku needs to be highlighted as it is perhaps the first time that the fusion of mathematics and materials science has been central. My collaboration continues with Miyazaki, whose insights into geometry are phenomenal. He generously gifted me several books, including his Adventure in Multidimensional Space: The Art and Geometry of Polygons, Polyhedra and Polytopes, which has been an important influence in my career. Since Japan is the foreign country I have visited the most – over 20 times – the publication of this new edition is an extremely pleasing development and cements my relationship with Japan and Japanese scientists. Its title in Japanese is “Solid Geometry for the Microcosm”.
It seems appropriate that while the earlier editions of the book appeared in the Heisei era, the translation into Japanese appears in the Reiwa era which has just dawned, and stands for harmony and beauty.
(As told to Deepika S)
Srinivasa Ranganathan is a metallurgist and former Chair of the Department of Materials Engineering and the Division of Mechanical Sciences, IISc, and Honorary Homi Bhabha Chair at the National Institute of Advanced Studies.